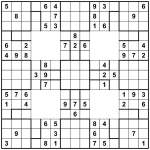
Gap Sudoku
Jigsaw Gap Samurai
Apr 12th
This is an interesting puzzle! It’s similar to yesterday’s Gap Samurai puzzles, except that the 3×3 box regions have been replaced by jigsaw regions – and not only that, but also some of these regions stretch across the empty areas of the puzzle.
The rules are:
- Wherever you see a continuous row or column of 9 squares from a bold line to a bold line then you must place 1 to 9.
- Rows or columns that cross gaps have no restrictions (numbers can repeat on the other side of the gap).
- Jigsaw regions must also have 1 to 9 in. Those jigsaw regions without bold lines next to a gap continue on the other side of the gap, by following a direct line across the gap. They do not flow around to the left or right, but only straight across. (If you’re familiar with Toroidal Sudoku, the regions connect in a similar way, except without actually wrapping around the outside of the puzzle too).
Confused? It isn’t actually that complex in concept, but keeping track of all the regions when solving might require a clear head! You might find it easier if you lightly colour in the different cross-gap regions in different colours in order to help keep track of them. (Sorry I haven’t coloured the PDF – I eventually will for future puzzles!)
If you’re still confused, and to clarify the regions further, count 4 across and 2 squares down from the top-left of the puzzle. This jigsaw region continues into the square below (obviously) and then across the gap to the square four below that (i.e. cross the gap whilst staying in the same column). It does not continue around the corner into the square that is 3 across by 4 down from the top-left – that’s part of a different region that continues in the centre of the puzzle (where the ‘8′ is, 8 across by 4 down). Returning to the first region, it then continues down to the ‘3′, and the ‘9′ and blank square to its right, and then down to the next square, across that second vertical gap, and then finishes in the two squares directly below (so that’s 4 across and 2/3 up from the bottom-left corner).
Phew! Good luck!
PS None of the Samurai puzzles I’m posting require complex solving logic – just an organised approach! (So you don’t need to consider naked or hidden sets, or anything more complex, although of course they might occasionally help anyway – but you can solve these puzzles without them).
PPS If you want to see solutions for any puzzles, just post a comment and ask! Also if you’ve solved one, please let me know how long it took – I’m interested to know!
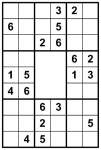
Gap Samurai Sudoku
Apr 11th
I thought it would be interesting to try creating Samurai puzzles with gaps in, but to do this not just by creating an enormous puzzle but by putting gaps actually into the underlying Sudoku grids, so some rows and columns are incomplete.
Take a look at the 6×9 puzzle attached (”2-grid 6×6 Samurai Gap puzzle”). Can you place 1 to 6 into each unbroken row and each bold-lined 2×3 box, whilst placing 1 to 6 into the top 6 and bottom 6 squares in each unbroken column? In other words, there are no rows or columns where there is a gap in the puzzle – you can repeat numbers on opposite sides of the gap.
This smaller puzzle is actually very easy, so I tried making a larger one with four 3×3 boxes ‘punched out’ of the puzzle. Again, where rows/columns run across the gaps then there are no restrictions on numbers repeating. Everywhere you find a continuous run of 9 squares from a bold line to a bold line in a row or a column then you must place all of 1-9 into that region. Also all of the 3×3 bold-lined boxes must contain 1 to 9 as usual.
In summary:
- Any continuous run of 9 squares starting on a bold line must have 1-9 in it, but
- Where a run of squares reaches a blank area then there are NO restrictions on that run – rows or columns don’t run across the gaps, in other words.
Incidentally, it would be perfectly reasonable for the rows/columns to continue across the gaps, but they don’t in these two puzzles – maybe they will in tomorrow’s puzzle! (it’s the obvious thing to change after all).


Recent Comments